题目
n 皇后问题 研究的是如何将 n 个皇后放置在 n × n 的棋盘上,并且使皇后彼此之间不能相互攻击。
给你一个整数 n ,返回 n 皇后问题 不同的解决方案的数量。
示例 1: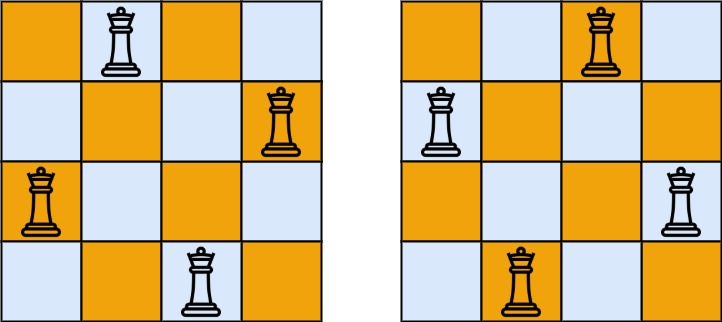
输入:n = 4
输出:2
解释:如上图所示,4 皇后问题存在两个不同的解法。1
2
3
2
3
示例 2:
输入:n = 1
输出:11
2
2
提示:
1 <= n <= 9
题解
java
public int totalNQueens(int n) {
List<Integer> result = new ArrayList<>();
result.add(0);
// 放置皇后置为1
List<StringBuilder> queens = new ArrayList<>();
for (int i = 0; i < n; i++) {
queens.add(new StringBuilder(String.join("", Collections.nCopies(n, "."))));
}
// 回溯找解
Consumer<Integer> solve = new Consumer<Integer>() {
@Override
public void accept(Integer index) {
if (index == n) {
result.set(0, result.get(0) + 1);
return;
}
for (int i = 0; i < n; i++) {
boolean found = false;
// 同一行存在皇后
if (queens.get(i).indexOf("Q") > -1) {
continue;
}
// 对角线上存在皇后
int j = i, k = index;
while (j >= 0 && k >= 0) {
if (queens.get(j--).charAt(k--) == 'Q') {
found = true;
break;
}
}
if (found) {
continue;
}
j = i;
k = index;
while (j < n && k >= 0) {
if (queens.get(j++).charAt(k--) == 'Q') {
found = true;
break;
}
}
if (found) {
continue;
}
// 拒绝同列出现多个皇后
queens.get(i).setCharAt(index, 'Q');
this.accept(index + 1);
// 回溯
queens.get(i).setCharAt(index, '.');
}
}
};
solve.accept(0);
return result.get(0);
}1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58